First order exponential smoothing

First order exponential smoothing or simple exponential smoothing is suitable with constant variance and no seasonality. The approach is usually recommended to make short-term forecast. Chapter 2, Understanding Time Series data, has introduced the naïve method for the forecasting where prediction in horizon h is defined as value of t (or the last observation):

x_t+h = x_t

The approach is extended by simple moving average, which extends the naïve approach using the mean of multiple historical points:

The approach assumes equal weight to all historical observations, as shown in the following figure:

Figure 3.4: Weight assigned to observation with increasing window size

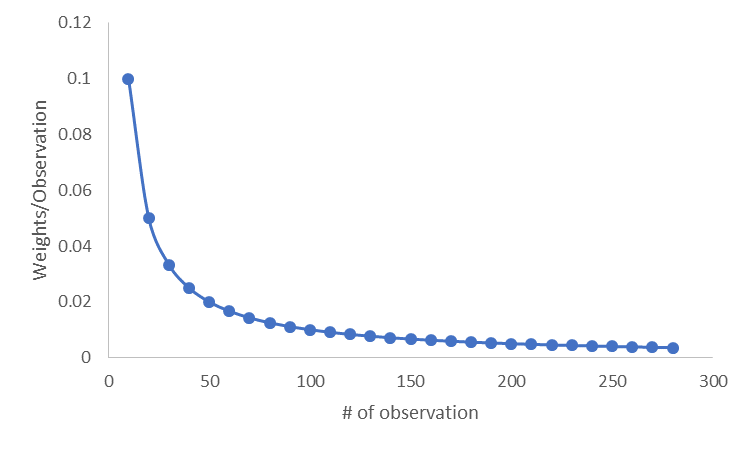
As the window size for moving average increases, the weights assigned to each observation become smaller. The first order exponential extends this current approach by providing...