Architecting the Mandelbrot project
The example project of this chapter is the multi-threaded calculation of a Mandelbrot fractal. The user will see the fractal and will be able to pan and zoom in that window.
Before diving into the code, we have to have a broad understanding of a fractal and how we are going to achieve its calculation.
The Mandelbrot fractal is a numerical set that works with complex numbers (a + bi). Each pixel is associated with a value calculated through iterations. If this iterated value diverges towards infinity then the pixel is out of the Mandelbrot set. If not, then the pixel is inside the Mandelbrot set. A visual representation of the Mandelbrot fractal looks like this:
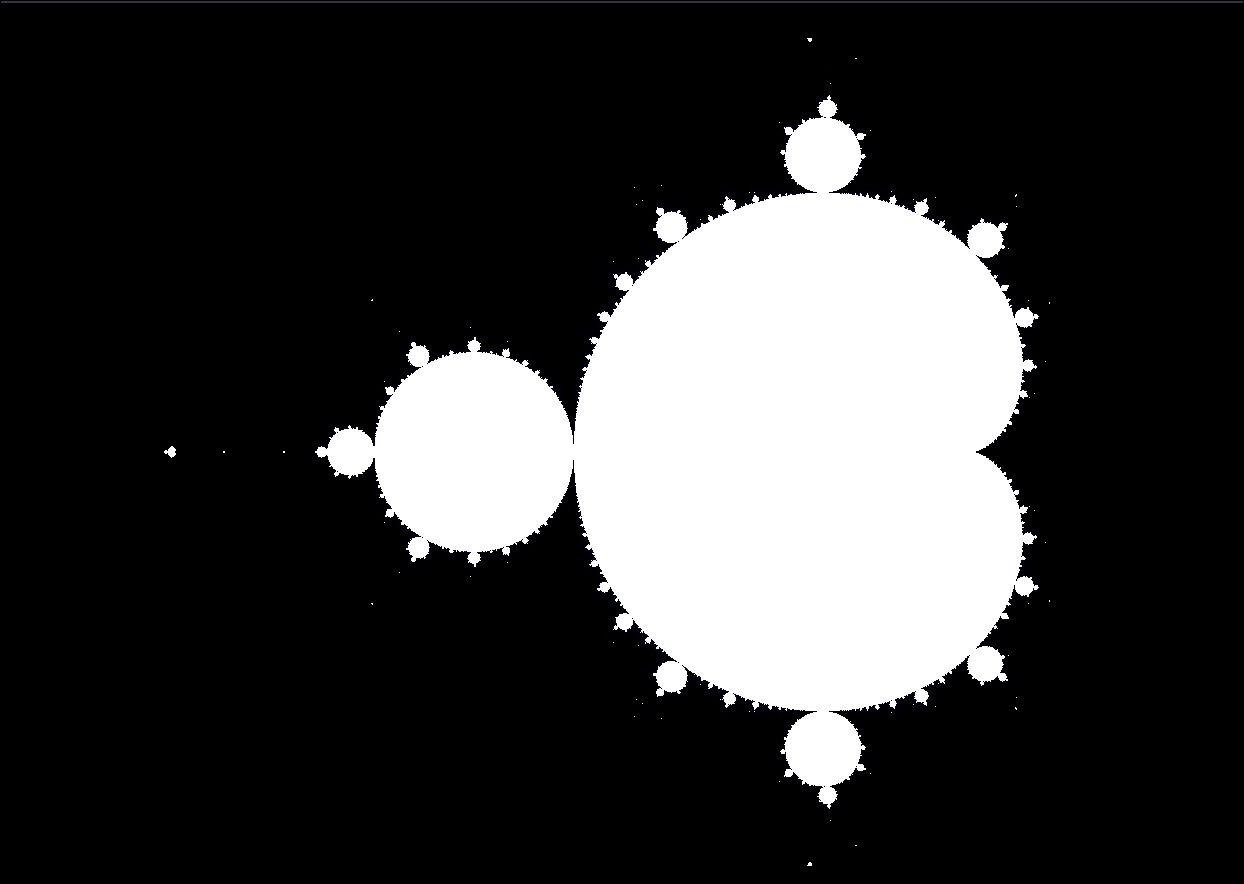
Every black pixel in this image tends to diverge to an infinite value, whereas white pixels are bounded to a finite value. The white pixels belong to the Mandelbrot set.
What makes it interesting from a multi-threaded perspective, is that to determine if the pixel belongs or not to the Mandelbrot set...
































































